1
Let  and fix
and fix 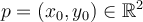 . Each of the derivatives stated in the below answer options is computed
at
. Each of the derivatives stated in the below answer options is computed
at  . Given these conditions, which of the statements
below is incorrect?
. Given these conditions, which of the statements
below is incorrect?
Choose one answer.
|
a. If the partial derivatives |
||
| b. The directional derivative, if it exists, is a finite real number. | ||
|
c. For all vectors |
||
|
d. If the restriction of |
Question
2
Let  be a function for which all directional
derivatives at all points exist. Define a new function
be a function for which all directional
derivatives at all points exist. Define a new function  by the formula
by the formula 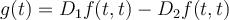 . Given these conditions,
which of the statements below is correct?
. Given these conditions,
which of the statements below is correct?
Choose one answer.
|
a. Regardless of what |
||
|
b. If |
||
|
c. The function |
||
|
d. If |
Question
3
Which of the statements below is incorrect?
Choose one answer.
|
a. The Jacobian of a function |
||
|
b. Any positive number can arise as the Jacobian at the origin of some function |
||
|
c. If |
||
|
d. If |
Question
4
Consider a function  and a point
and a point 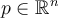 . Which of the statements below is correct?
. Which of the statements below is correct?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
5
Let  be a function satisfying the homogeneity
condition
be a function satisfying the homogeneity
condition 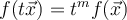 for some
for some 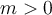 . Which of the statements below is correct?
. Which of the statements below is correct?
Choose one answer.
|
a. If |
||
|
b. |
||
|
c. |
||
|
d. If the homogeneity condition is satisfied, then |
Question
6
Consider the function  , given by
, given by  . Which of the statements below is incorrect?
. Which of the statements below is incorrect?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
7
For the functions 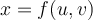 and
and 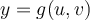 , which answer option below results in a Jacobian that is not independent
of
, which answer option below results in a Jacobian that is not independent
of 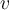 ?
?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
8
Let 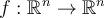 be a function that is continuously
differentiable at all points
be a function that is continuously
differentiable at all points  . Which of the
statements below is correct?
. Which of the
statements below is correct?
Choose one answer.
|
a. If at every point |
||
|
b. If at some point |
||
|
c. If the Jacobian of |
||
|
d. If the Jacobian of |
Question
9
For each positive real number 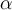 , let
, let 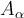 be a subset of
be a subset of 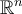 . Which of the statements below is incorrect?
. Which of the statements below is incorrect?
Choose one answer.
|
a. If each |
||
|
b. If for all |
||
|
c. If |
||
|
d. If |
Question
10
Let  be a function of class
be a function of class 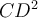 . Write
. Write 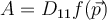 ,
,  , and
, and  . Given these conditions, which of the statements below is correct?
. Given these conditions, which of the statements below is correct?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
|
d. If |
Question
11
Let  be given and
be given and 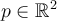 a point. Further, let
a point. Further, let 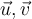 be vectors in
be vectors in 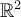 . All the derivatives in the below answer options are computed at
. All the derivatives in the below answer options are computed at
 . Without further knowledge about
. Without further knowledge about 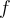 other than that given in each statement below, which of the answer
options below is correct?
other than that given in each statement below, which of the answer
options below is correct?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
| d. Since there are infinitely many directions in which to compute directional derivatives, knowledge of any finite number of directional derivatives is never sufficient to determine all directional derivatives. |
Question
12
Let 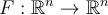 be a function whose component functions
be a function whose component functions
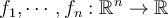 all have continuous partial derivatives. Which of
the statements below is correct?
all have continuous partial derivatives. Which of
the statements below is correct?
Choose one answer.
|
a. If |
||
|
b. If |
||
| c. The Jacobian is either always positive or always negative. | ||
|
d. If the Jacobian of |
Question
13
Which of the statements below is incorrect?
Choose one answer.
|
a. For every |
||
|
b. The set |
||
|
c. If |
||
| d. A closed set is never meager. |
Question
14
The method of Lagrange multipliers typically is used to:
Choose one answer.
| a. compute partial derivatives of a function conditioned on excluding particular directions. | ||
|
b. find the largest value of |
||
| c. find the inverse of a function when the Inverse Function Theorem applies but does not yield an unconditioned value. | ||
|
d. normalize the Jacobian, conditioned on the sign of the first |
Question
15
Let 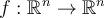 be given. Which of the statements below is
incorrect?
be given. Which of the statements below is
incorrect?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
|
d. The Jacobian of |
Question
16
Let  be given. Which of the statements below is
incorrect?
be given. Which of the statements below is
incorrect?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If any restriction of |
||
|
d. |
Question
17
Let  be given such that
be given such that 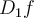 ,
, 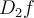 ,
, 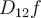 and
and 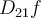 all exist at every point
all exist at every point  in the domain. Fix a point
in the domain. Fix a point 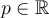 . Which of the below statements relating to the differentiability at this
point is correct?
. Which of the below statements relating to the differentiability at this
point is correct?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
18
Consider the function  given by the formula
given by the formula
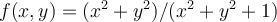 . Which of the statements below is incorrect?
. Which of the statements below is incorrect?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
19
Let 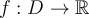 be defined on some subset
be defined on some subset  . Assume that
. Assume that 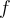 is differentiable at a given point
is differentiable at a given point  in
in 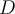 . Which of the statements below is incorrect?
. Which of the statements below is incorrect?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
|
d. If |
Question
20
Which of the statements below is incorrect?
Choose one answer.
|
a. If |
||
|
b. The theorem that a function |
||
|
c. For every |
||
|
d. If |
Question
21
Let 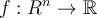 be a function, and
be a function, and  a rectangle. Which of the statements below is incorrect?
a rectangle. Which of the statements below is incorrect?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
|
d. If |
Question
22
Which of the statements below is incorrect?
Choose one answer.
| a. The set of all rational numbers is a set of zero content. | ||
| b. The set of all irrational numbers is a set of zero content. | ||
| c. A countable union of sets of zero content is itself a set of zero content. | ||
| d. Any finite set is a set of zero content. |
Question
23
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If the set |
||
|
d. If |
Question
24
Which of the following functions 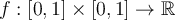 is not Riemann
integrable?
is not Riemann
integrable?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
25
Let  , with
, with  . Which of the statements below is correct?
. Which of the statements below is correct?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If for all |
||
|
d. If |
Question
26
Let 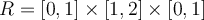 and
and  . What can be said about
. What can be said about 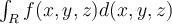 ?
?
Choose one answer.
| a. The integral does not exist. | ||
|
b. The integral exists and equals |
||
|
c. The integral exists and equals |
||
| d. The integral exists, but its value can't be evaluated in closed form. |
Question
27
Let  be a linear transformation, and let
be a linear transformation, and let
 . Which of the statements below is correct?
. Which of the statements below is correct?
Choose one answer.
|
a. The Jordan content of |
||
|
b. If |
||
|
c. If |
||
|
d. The Jordan content of |
Question
28
The answer options below are concerned with subsets of 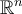 . Which of the statements below is incorrect?
. Which of the statements below is incorrect?
Choose one answer.
| a. The union of finitely many Jordan measurable subsets is itself Jordan measurable. | ||
| b. The intersection of a Jordan measurable subset and a set of zero content is itself a set of zero content. | ||
|
c. If |
||
|
d. If |
Question
29
Let 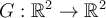 and
and  . Assuming that the integrals below exist, under which of the conditions
below is the formula
. Assuming that the integrals below exist, under which of the conditions
below is the formula 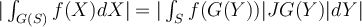 guaranteed to hold?
guaranteed to hold?
Choose one answer.
|
a. If |
||
|
b. If |
||
| c. The stated equality never holds. | ||
| d. The stated equality always holds. |
Question
30
Let 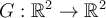 be a linear transformation with determinant
be a linear transformation with determinant
 . Let
. Let  ,
, 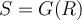 , and
, and 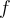 be Riemann integrable on a rectangle containing
be Riemann integrable on a rectangle containing 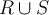 . Which of the statements below is necessarily correct?
. Which of the statements below is necessarily correct?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
31
Let 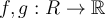 , where
, where  , be Riemann integrable. Assume that for all
, be Riemann integrable. Assume that for all  , it holds that
, it holds that 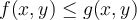 . Which of the following statements is true?
. Which of the following statements is true?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
| d. All of the answer options above hold true. |
Question
32
Let 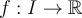 , where
, where 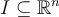 is some non-degenerate rectangle (i.e., it has positive
is some non-degenerate rectangle (i.e., it has positive 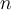 -dimensional volume). Which of the following statements is correct?
-dimensional volume). Which of the following statements is correct?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
| d. All of the answer options above are correct. |
Question
33
Let 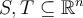 . Which of the statements below is incorrect?
. Which of the statements below is incorrect?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
|
d. If |
Question
34
Consider the region 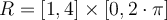 . The polar coordinate
transformation
. The polar coordinate
transformation 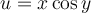 ,
,  achieves which of the following?
achieves which of the following?
Choose one answer.
|
a. It transforms the region |
||
|
b. It transforms the region |
||
|
c. It transforms the region |
||
|
d. It transforms the region |
Question
35
Which of the following functions 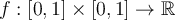 is Riemann
integrable?
is Riemann
integrable?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
| d. All of the answer options above are Riemann integrable functions. |
Question
36
Let 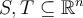 be Jordan measurable, and
be Jordan measurable, and 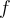 be a function that is integrable on both
be a function that is integrable on both 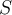 and
and 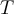 . Which of the statements below is correct?
. Which of the statements below is correct?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
|
d. If |
Question
37
Which of the following subsets 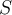 of
of 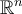 , with
, with 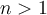 , is of zero content?
, is of zero content?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
| d. All of the answer options above describe sets of zero content. |
Question
38
Let 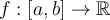 and
and 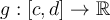 be continuous functions. Let
be continuous functions. Let 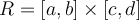 . Assume that
. Assume that 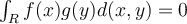 . Which of the statements below is correct?
. Which of the statements below is correct?
Choose one answer.
|
a. Both |
||
|
b. Either |
||
|
c. For all |
||
|
d. For all |
Question
39
Let 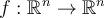 . Which of the following statements is
incorrect?
. Which of the following statements is
incorrect?
Choose one answer.
|
a. There exists an infinite subset |
||
|
b. If |
||
|
c. If |
||
|
d. If |
Question
40
Let 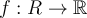 where
where 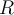 is a non-trivial rectangle in
is a non-trivial rectangle in 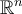 . Which of the statements below is correct?
. Which of the statements below is correct?
Choose one answer.
|
a. If |
||
|
b. |
||
|
c. If |
||
|
d. If |
Question
41
Which of the following answer options is a  -form on
-form on
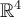 ?
?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
42
Let 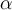 be a
be a 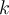 -form on
-form on 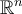 . Which of the following statements is correct?
. Which of the following statements is correct?
Choose one answer.
|
a. |
||
|
b. If |
||
|
c. If |
||
|
d. |
Question
43
Let 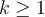 and
and 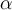 be a
be a 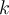 -form on
-form on 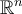 . Which of the following statements is incorrect?
. Which of the following statements is incorrect?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
44
In 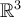 , which of the following statements is correct?
, which of the following statements is correct?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
45
Let 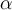 be a
be a 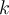 -form on
-form on 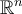 , with
, with 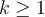 and
and 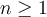 . Which of the following statements is correct?
. Which of the following statements is correct?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
|
d. The validity or invalidity of each answer option above depends on the values of |
Question
46
Consider 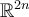 with coordinates
with coordinates 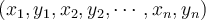 . Let
. Let 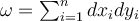 , and let
, and let  denote the volume form on
denote the volume form on 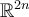 . Which of the following statements is correct?
. Which of the following statements is correct?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
47
Let 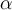 be the form
be the form 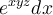 . Which of the following answer options is equal to
. Which of the following answer options is equal to 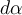 ?
?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
48
Let 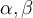 be
be 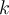 -forms on
-forms on 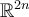 . Which of the following statements is correct?
. Which of the following statements is correct?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. If |
||
|
d. If |
Question
49
Let 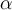 and
and  be differential forms on
be differential forms on 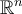 . Which of the following statements is incorrect?
. Which of the following statements is incorrect?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
|
d. If |
Question
50
Let 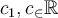 be constants. Consider the form
be constants. Consider the form  . For which of the following answer options is
. For which of the following answer options is 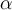 closed?
closed?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
| d. All of the answer options above lead to a closed form. |
Question
51
Let 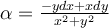 and
and  be the unit circle curve, parametrized counterclockwise. What is the
value of
be the unit circle curve, parametrized counterclockwise. What is the
value of 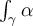 ?
?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
52
Let 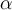 be a
be a 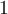 -form on
-form on 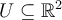 and let
and let 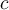 be a curve for which
be a curve for which 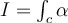 exists. Which of the following statements is correct?
exists. Which of the following statements is correct?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
|
d. If |
Question
53
Consider a closed curve  satisfying
satisfying 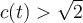 for all
for all 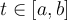 , and let
, and let 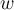 be the winding number of
be the winding number of 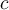 . Which of the following statements is correct?
. Which of the following statements is correct?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
|
d. |
Question
54
Let 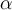 be a
be a 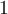 -form on
-form on 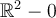 and let
and let 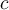 be a curve that does not pass through the origin. Which of the following
statements is correct?
be a curve that does not pass through the origin. Which of the following
statements is correct?
Choose one answer.
|
a. If |
||
|
b. If |
||
|
c. If |
||
| d. All of the above answer options are correct. |
Question
55
Which of the following statements regarding chains, boundaries, cycles, and forms is correct?
Choose one answer.
|
a. If |
||
|
b. The boundary of a degenerate |
||
| c. Every boundary is a cycle. | ||
| d. All of the above statements are correct. |
Question
56
Which of the following statements regarding chains and boundaries is correct?
Choose one answer.
| a. Every cycle is a boundary. | ||
|
b. |
||
| c. A chain is a boundary if, and only if, it is a cycle. | ||
| d. All of the statements above are incorrect. |
Question
57
Let 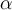 be a
be a 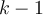 -form on an open set
-form on an open set  and let
and let 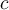 be a
be a 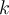 -chain. Which of the following equalities is guaranteed to hold?
-chain. Which of the following equalities is guaranteed to hold?
Choose one answer.
|
a. |
||
|
b. |
||
|
c. |
||
| d. None of the above equalities is correct. |
Question
58
When the most general Stokes' Theorem is applied to the 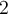 -form
-form 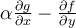 to compute the integral
to compute the integral 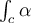 , where
, where 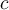 is a smooth curve in
is a smooth curve in 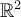 enclosing a region in which
enclosing a region in which 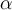 is defined, what is the resulting formula's name?
is defined, what is the resulting formula's name?
Choose one answer.
| a. Stokes' Formula. | ||
| b. Green's Formula. | ||
| c. Gauss' Formula. | ||
| d. The resulting formula has no particular name. |
Question
59
The determinant of an 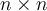 matrix whose columns form a
linearly dependent set is known to be
matrix whose columns form a
linearly dependent set is known to be 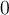 . Which of the
following statements is a correct geometric explanation for this fact?
. Which of the
following statements is a correct geometric explanation for this fact?
Choose one answer.
|
a. Such a matrix represents a linear transformation whose values are independent of a particular choice of
coordinates. Since volume depends on coordinates, the determinant must be |
||
|
b. Such a matrix represents a linear transformation whose values are dependent on a particular choice of basis.
Since volume depends on the basis, the determinant must be |
||
|
c. The |
||
| d. All of the above statements are correct geometric explanations. |
Question
60
The pullback operator connects which of the following two concepts?
Choose one answer.
| a. The global angle form and the gradient. | ||
| b. The global angle form and the Jacobian. | ||
| c. The volume forms and the gradient. | ||
| d. The volume forms and the Jacobian. |