Chapter 7 Externalities

7.1 External Effects

These effects are called external effects, or externalities. An externalityAny effect on people not involved in a particular transaction. is any effect on people not involved in a particular transaction. Pollution is the classic example. When another person buys and smokes cigarettes, there is a transaction between the cigarette company and the smoker. But if you are sitting near the smoker, you are an affected party who is not directly compensated from the transaction, at least before taxes were imposed on cigarettes. Similarly, you pay nothing for the benefits you get from viewing your neighbor’s flowers, nor is there a direct mechanism to reward your neighbor for her efforts.

Externalities will generally cause competitive markets to behave inefficiently from a social perspective, absent a mechanism to involve all the affected parties. Without such a mechanism, the flower planter will plant too few beautiful flowers, for she has no reason to take account of your preferences in her choices. The odious smoker will smoke too much and too close to others, and the loud neighbor will play music much too late into the night. Externalities create a market failureA situation where a competitive market does not yield the socially efficient outcome.—that is, a situation where a competitive market does not yield the socially efficient outcome.

Education is viewed as creating an important positive externality. Education generates many externalities, including more—and better—employment, less crime, and fewer negative externalities of other kinds. It is widely believed that educated voters elect better politicians.This is a logical proposition, but there is scant evidence in favor of it. There is evidence that educated voters are more likely to vote, but little evidence that they will vote for better candidates. Educated individuals tend to make a society wealthy, an advantage to all of society’s members. As a consequence, most societies subsidize education in order to promote it.

A major source of externalities arises in communicable diseases. Your vaccination not only reduces the likelihood that you will contract a disease but also makes it less likely that you will infect others with the disease.

Let’s consider pollution as a typical example. A paper mill produces paper, and a bad smell is an unfortunate by-product of the process. Each ton of paper produced increases the amount of bad smells produced. The paper mill incurs a marginal cost, associated with inputs like wood and chemicals and water. For the purposes of studying externalities, we will refer to the paper mill’s costs as a private costThe cost borne by the supplier., the cost borne by the supplier (in this case, the paper mill itself). In addition, there are external costsThe costs borne by third parties., which are the costs borne by third parties, that arise in this case from the smell. Adding the private costs and the external costs yield the total costs for all parties, or the social costsThe total costs for all parties.. These costs, in their marginal form, are illustrated in Figure 7.1 "A negative externality".

In Figure 7.1 "A negative externality", the demand has been labeled “marginal benefit,” for reasons that will become apparent; but it is at this point just the standard demand, the marginal value of the product. The paper mill’s costs have been labeled marginal private cost to reflect the fact that these costs are only the mill’s costs and don’t include the cost of the bad smell imposed on others. The marginal social cost is obtained by adding the marginal external cost to the marginal private cost. The marginal external cost isn’t graphed in the figure; but the size of it is illustrated at one quantity, and it is generally the difference between marginal social cost and marginal private cost.

Left to its own devices, the paper market would equate the marginal private cost and the marginal benefit to produce the competitive quantity sold at the competitive price. Some of these units—all of those beyond the quantity labeled “Socially Efficient Quantity”—are bad from a social perspective: They cost more to society than they provide in benefits. This is because the social cost of these units includes pollution, but paper buyers have no reason to worry about pollution or even to know that it is being created in the process of manufacturing paper.

The deadweight loss of these units is shown as a shaded triangle in the figure. The loss arises because the marginal social cost of the units exceeds the benefit, and the difference between the social cost and the benefits yields the loss to society. This is a case where too much is produced because the market has no reason to account for all the costs; some of the costs are borne by others.

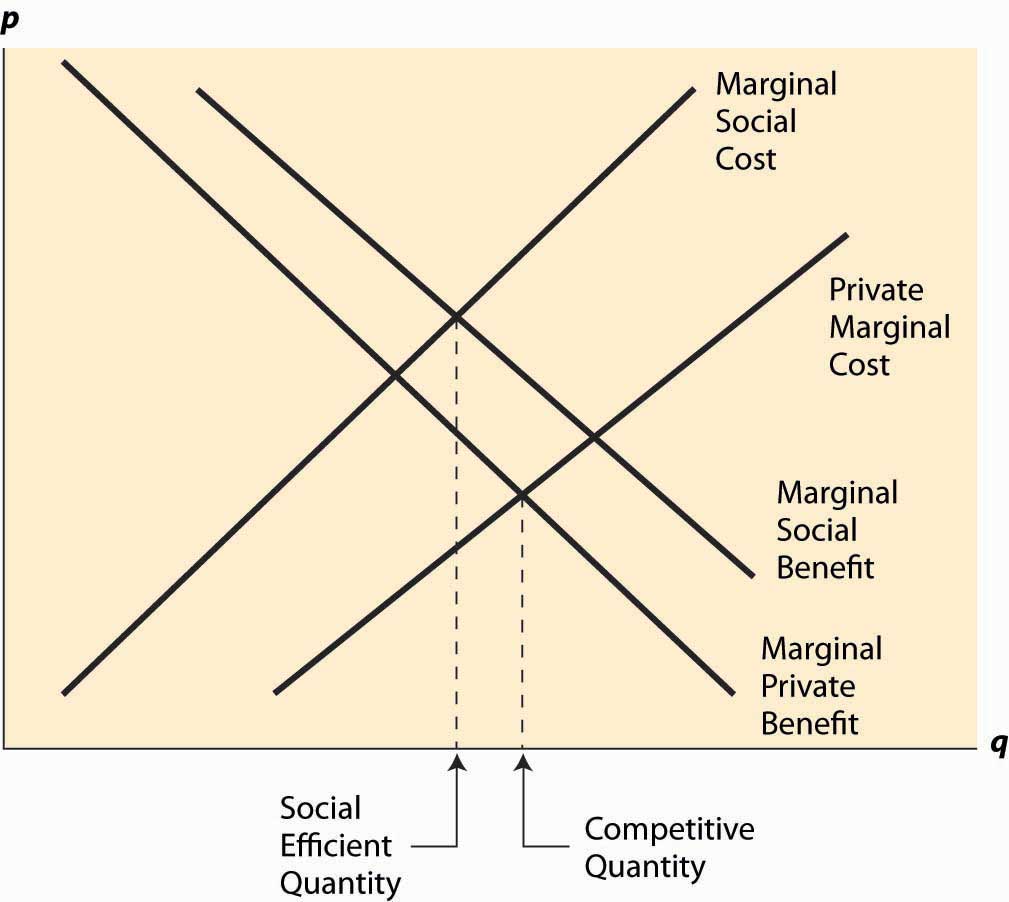
Generally, a negative externality like pollution creates a marginal social cost that is higher than the marginal private cost. Similarly, a positive externality like beautification creates a higher marginal social benefitA benefit for all parties.—a benefit for all parties—than the marginal private benefit (demand), with the difference being benefits obtained by third parties, or external benefitsBenefits obtained by third parties.. These are to some extent conventions. One could have incorporated a positive externality by a reduction in cost—but the convention remains. An example of a product that produces both positive and negative externalities is illustrated in Figure 7.2 "External costs and benefits". Streetlights are an example of a product that produces both externalities: Most of us like lighted streets, but they are terrible for astronomers. Similarly, large highways produce benefits for commuters and yet harm nearby residents.

The marginal private benefitThe benefit obtained by the buyer.—the benefit obtained by the buyer—and the marginal private cost give the demand and supply of a competitive market, and hence the competitive quantity results from the intersection of these two. The marginal social benefit and the marginal social cost give the value and cost from a social perspective; equating these two generates the socially efficient outcome. This can be either greater or less than the competitive outcome depending on which externality is larger.

Consider a town on a scenic bay that is filled with lobsters. The town members collect and eat the lobsters, and over time the size of the lobsters collected falls, until they are hardly worth searching for. This situation persists indefinitely. Few large lobsters are caught, and it is barely worth one’s time attempting to catch them. This sort of overuse of a resource due to lack of ownership is known as the tragedy of the commonsOveruse of a resource due to lack of ownership..

The tragedy of the commons is a problem with a common resourceA resource shared by many people. shared by many people—in this case, the lobster bay. Catching lobsters creates an externality by lowering the productivity of other lobster catchers. The externality leads to overfishing, since individuals don’t take into account the negative effect they have on each other, ultimately leading to a nearly useless resource and potentially driving the lobsters to extinction. As a consequence, the lobster catching is usually regulated.

7.2 Pigouvian Taxes

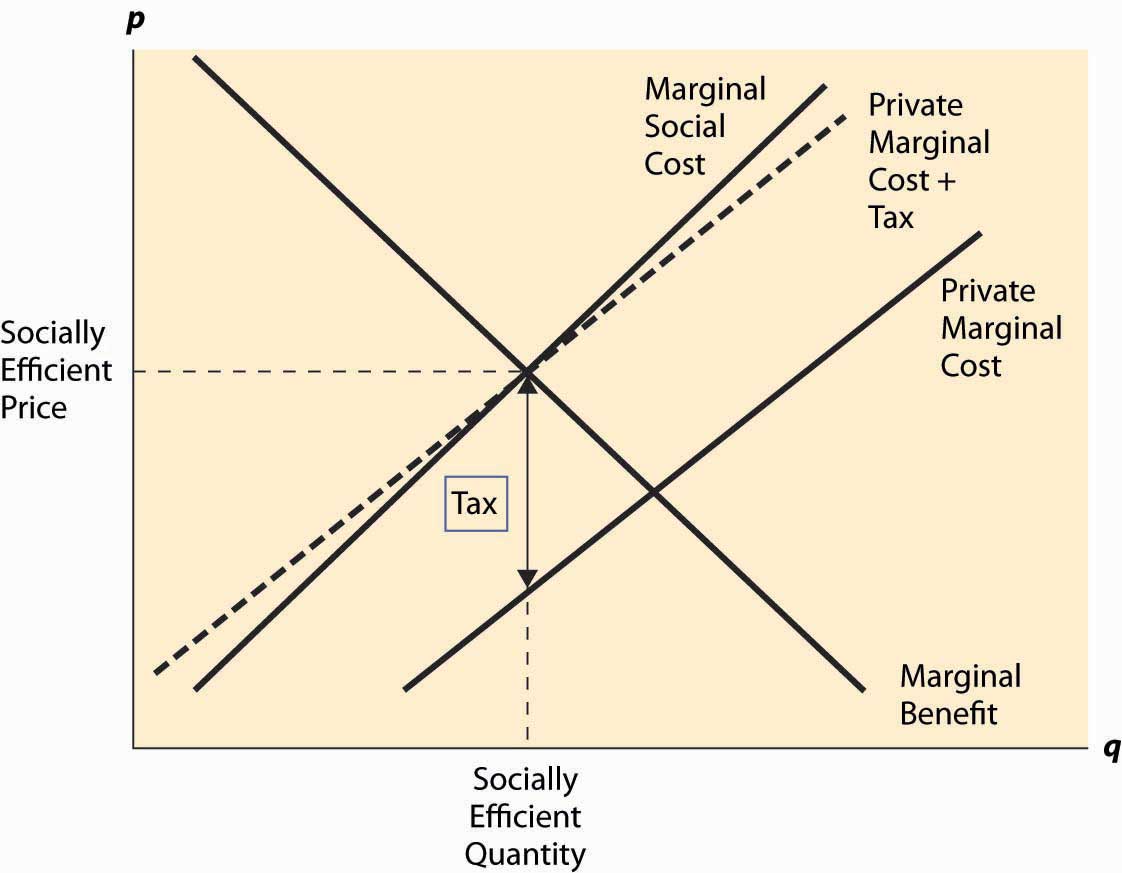
Arthur Cecil Pigou (1877–1959) proposed a solution to the problem of externalities that has become a standard approach. This simple idea is to impose a per-unit tax on a good, thereby generating negative externalities equal to the marginal externality at the socially efficient quantity. This is known as a Pigouvian taxA per-unit tax on a good.. Thus, if at the socially efficient quantity, the marginal external cost is $1, then a $1 per-unit tax would lead to the right outcome. This is illustrated in Figure 7.3 "The Pigouvian tax".

The tax that is added is the difference, at the socially efficient quantity, between the marginal social cost and the marginal private cost, which equals the marginal external cost. The tax level need not equal the marginal external cost at other quantities, and the figure reflects a marginal external cost that is growing as the quantity grows. Nevertheless, the new supply curve created by the addition of the tax intersects demand (the marginal benefit) at the socially efficient quantity. As a result, the new competitive equilibrium, taking account of the tax, is efficient.

The case of a positive externality is similar. Here, a subsidy is needed to induce the efficient quantity. It is left as an exercise.

Taxes and subsidies are fairly common instruments to control externalities. We subsidize higher education with state universities, and the federal government provides funds for research and limited funds for the arts. Taxes on cigarettes and alcoholic beverages are used to discourage these activities, perhaps because smoking and drinking alcoholic beverages create negative externalities. (Cigarettes and alcohol also have inelastic demands, which make them good candidates for taxation since there is only a small distortion of the quantity.) However, while important in some arenas, taxes and subsidies are not the most common approach to regulation of externalities.

7.3 Quotas

The Pigouvian tax and subsidy approach to dealing with externalities has several problems. First, it requires knowing the marginal value or cost of the external effect, and this may be a challenge to estimate. Second, it requires the imposition of taxes and permits the payment of subsidies, which encourages what might be politely termed as “misappropriation of funds.” That is, once a government agency is permitted to tax some activities and subsidize others, there will be a tendency to tax things people in the agency don’t like and subsidize “pet” projects, using the potential for externalities as an excuse rather than a real reason. U.S. politicians have been especially quick to see positive externalities in oil, cattle, and the family farm—externalities that haven’t been successfully articulated. (The Canadian government, in contrast, sees externalities in filmmaking and railroads.)

An alternative to the Pigouvian tax or subsidy solution is to set a quota, which is a limit on the activity. Quotas can be maxima or minima, depending on whether the activity generates negative or positive externalities. We set maximum levels on many pollutants rather than tax them, and ban some activities, like lead in gasoline or paint, or chlorofluorocarbons outright (a quota equal to zero). We set maximum amounts on impurities, like rat feces, in foodstuffs. We impose minimum educational attainment (eighth grade or age 16, whichever comes first), minimum age to drive, and minimum amount of rest time for truck drivers and airline pilots. A large set of regulations governs electricity and plumbing, designed to promote safety, and these tend to be “minimum standards.” Quotas are a much more common regulatory strategy for dealing with externalities than taxes and subsidies.

The idea behind a quota is to limit the quantity to the efficient level. If a negative externality in pollution means our society pollutes too much, then impose a limit or quantity restriction on pollution. If the positive externality of education means individuals in our society receive too little education from the social perspective, force them to go to school.

As noted, quotas have the advantage of addressing the problem without letting the government spend more money, limiting the government’s ability to misuse funds. On the other hand, quotas have the problem of identifying who should get the quota; quotas will often misallocate the resource. Indeed, a small number of power plants account for almost half of the man-made sulfur dioxide (SO₂) pollution emitted into the atmosphere, primarily because these plants historically emitted a lot of pollution and their pollution level was set by their historical levels. Quotas tend to harm new entrants compared to existing firms and discourage the adoption of new technology. Indeed, the biggest polluters must stay with old technology in order to maintain their right to pollute.

7.4 Tradable Permits and Auctions

A solution to inefficiencies in the allocation of quota rights is to permit trading them. Tradable permitsQuotas for pollution that can be exchanged to create a market in the right to pollute. are quotas for pollution that can be exchanged to create a market in the right to pollute, and thereby create a tax on polluting. The emission of pollution requires the purchase of permits to pollute, and the price of these permits represents a tax on pollution. Thus, tradable permits represent a hybrid of a quota system and a Pigouvian taxation system—a quota determines the overall quantity of pollution as in a quota system, determining the supply of pollution rights, but the purchase of pollution rights acts like a tax on pollution, a tax whose level is determined by the quota supply and demand.

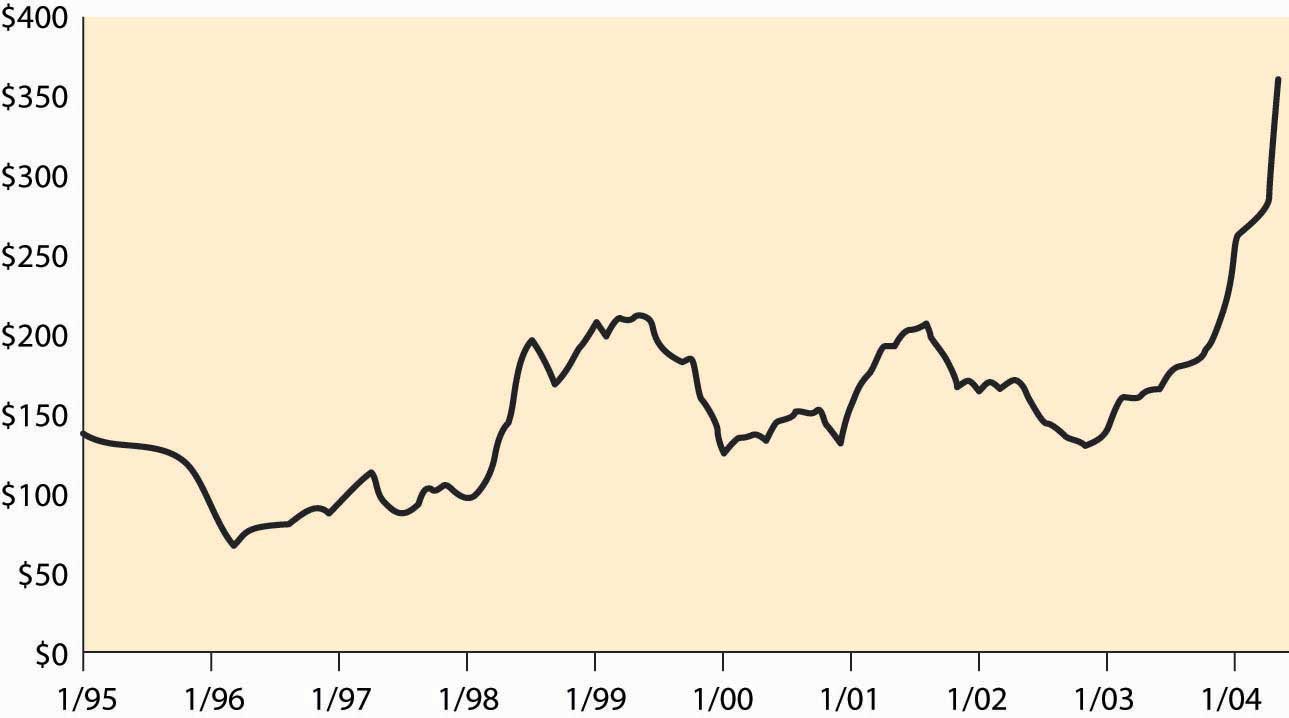
The United States has allowed the trading of permits for some pollutants, like sulfur dioxide. Figure 7.4 "SO" shows the price of sulfur dioxide permits over the past decade.Source: Environmental Protection Agency, July 22, 2004, http://www.epa.gov/airmarkets/trading/so2market/alprices.html Each permit conveys the right to emit one ton of sulfur dioxide into the air. The overall pollution level is being reduced over time, which accounts for some of the increase in prices. These prices represent significant taxes on large polluters, as a coal-fired power plant using coal with high sulfur content can annually produce as much as 200,000 tons of sulfur dioxide.

The major advantage of a tradable permits system is that it creates the opportunity for efficient exchange—one potential polluter can buy permits from another, leaving the total amount of pollution constant. Such exchange is efficient because it uses the pollution in a manner creating the highest value, eliminating a bias toward “old” sources. Indeed, a low-value polluter might sell its permits and just shut down if the price of pollution was high enough.

A somewhat unexpected advantage of tradable permits has been the purchase of permits by environmental groups like the Sierra Club. Environmental groups can buy permits and then not exercise them, as a way of cleaning the air. In this case, the purchase of the permits creates a major positive externality on the rest of society, since the environmental group expends its own resources to reduce pollution of others.

Tradable permits offer the advantages of a taxation scheme—efficient use of pollution—without needing to estimate the social cost of pollution directly. This is especially valuable when the strategy is to set a quantity equal to the current quantity, and then gradually reduce the quantity in order to reduce the effects of the pollution. The price of permits can be a very useful instrument in assessing the appropriate time to reduce the quantity, since high permit prices, relative to likely marginal external costs, suggest that the quantity of the quota is too low, while low prices suggest that the quantity is too large and should be reduced.

7.5 Coasian Bargaining

The negative externality of a neighbor playing loud music late at night is not ordinarily solved with a tax or with a quota but instead through an agreement. When there aren’t many individuals involved, the individuals may be able to solve the problem of externalities without involving a government but through negotiation. This insight was developed by Nobel laureate Ronald Coase (1910– ), and is sometimes known as Coasian bargainingSolving the problem of externalities through negotiation..

Coase offered the example of a cattle ranch next to a farm. There is a negative externality in that the cattle tend to wander over to the farm and eat the crops, rather than staying on the ranch. What happens next depends on property rightsRights that come with ownership., which are the rights that come with ownership.

One of three things might be efficient from a social perspective. It might be efficient to erect a fence to keep the cows away from the crops. It might be efficient to close down the farm. Finally, it might be efficient to close down the ranch, if the farm is valuable enough and if the fence costs more than the value of the ranch.

If the farmer has a right not to have his crops eaten and can confiscate the cows if they wander onto the farm, then the rancher will have an incentive to erect a fence to keep the cows away, if that is the efficient solution. If the efficient solution is to close down the ranch, then the rancher will do that, since the farmer can confiscate the cows if they go over to the farm and it isn’t worth building the fence by hypothesis. Finally, if the efficient solution to the externality is to close down the farm, then the rancher will have an incentive to buy the farm in order to purchase the farm’s rights so that he can keep the ranch in operation. Since it is efficient to close down the farm only if the farm is worth less than the ranch, there is enough value in operating the ranch to purchase the farm at its value and still have money left over; that is, there are gains from trade from selling the farm to the rancher. In all three cases, if the farmer has the property rights, then the efficient outcome is reached.

Now suppose instead that the rancher has the rights and that the farmer has no recourse if the cows eat his crops. If shutting down the farm is efficient, then the farmer has no recourse but to shut it down. Similarly, if building the fence is efficient, then the farmer will build the fence to protect his crops. Finally, if shutting down the ranch is efficient, the farmer will buy the ranch from the rancher in order to be able to continue to operate the more valuable farm. In all cases, the efficient solution is reached through negotiation.

Coase argued that bargaining can generally solve problems of externalities and that the real problem is ill-defined property rights. If the rancher and the farmer can’t transfer their property rights, then the efficient outcome may not arise. In the Coasian view of externalities, if an individual owned the air, air pollution would not be a problem because the owner would charge for the use and wouldn’t permit an inefficient level of pollution. The case of air pollution demonstrates some of the limitations of the Coasian approach because ownership of the air, or even the more limited right to pollute into the air, would create an additional set of problems; a case where the cure is likely to be worse than the disease.

Bargaining to solve the problem of externalities is often feasible when a small number of people are involved. When a large number of people are potentially involved, as with air pollution, bargaining is unlikely to be successful in addressing the problem of externalities, and a different approach is required.

7.6 Fishing and Extinction

Consider an unregulated fishing market like the lobster market considered previously, and let S be the stock of fish. The purpose of this example is illustrative of the logic, rather than an exact accounting of the biology of fish populations, but is not unreasonable. Let S be the stock of a particular species of fish. Our starting point is an environment without fishing: How does the fish population change over time? Denote the change over time in the fish population by $\dot{S}$ ($\dot{S}$ is notation for the derivative with respect to time, notation that dates back to Sir Isaac Newton). We assume that population growth follows the logistic equation $\dot{S}=rS(1-S).$ This equation reflects two underlying assumptions. First, mating and reproduction are proportional to the stock of fish S. Second, survival is proportional to the amount of available resources 1 – S, where 1 is set to be the maximum sustainable population. (Set the units of the number of fish so that 1 is the full population.)

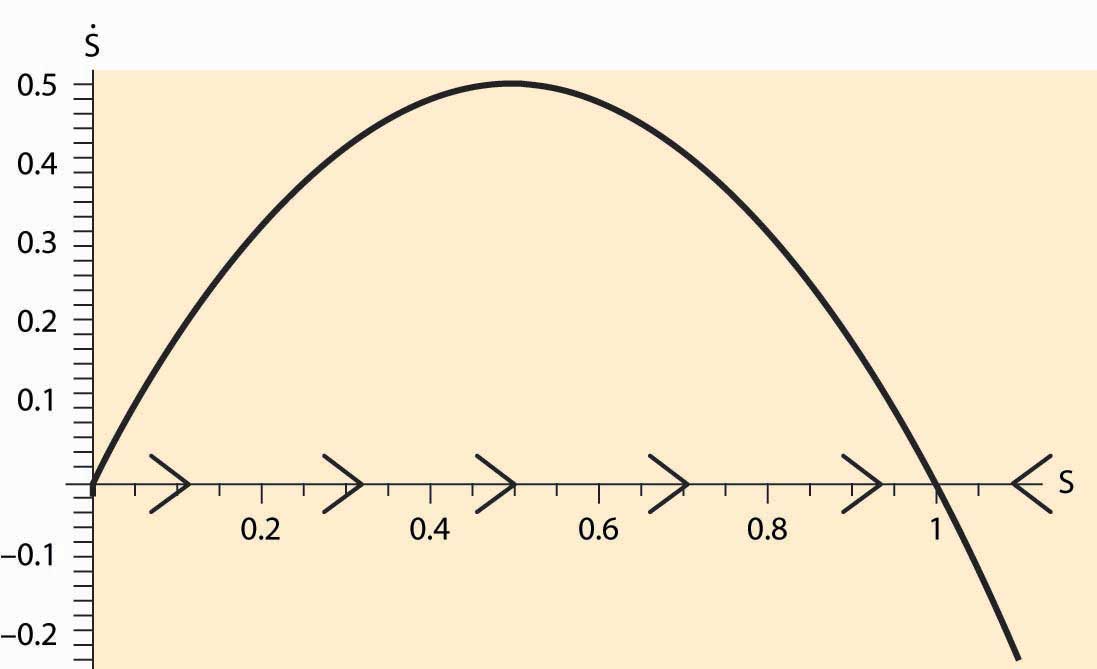
The dynamics of the number of fish are illustrated in Figure 7.5 "Fish population dynamics". On the horizontal axis is the number of fish, and on the vertical axis is the change in S. When $\dot{S}$ > 0, S is increasing over time, and the arrows on the horizontal axis reflect this. Similarly, if $\dot{S}$ < 0, S is decreasing.

Absent fishing, the value 1 is a stable steady stateCondition in which the variables stay constant and forces are balanced. of the fish population, in which the variables stay constant and forces are balanced. It is a steady state because, if S = 1, $\dot{S}$ = 0; that is, there is no change in the fish population. It is stable because the effect of a small perturbation—S near but not exactly equal to 1—is to return to 1. (In fact, the fish population is very nearly globally stable. Start with any population other than zero and the population returns to 1.)It turns out that there is a closed form solution for the fish population: $S(t)=\frac{S(0)}{S(0)+(1-S(0))e^{-rt}}\text{.}$

Now we introduce a human population and turn to the economics of fishing. Suppose that a boat costs b to launch and operate and that it captures a fixed fraction a of the total stock of fish S; that is, each boat catches aS. Fish sell for a price $p=Q^{\raisebox{1ex}{$-1$}\!\left/ \!\raisebox{-1ex}{$\epsilon $}\right.}\text{,}$ where the price arises from the demand curve, which in this case has constant elasticity ε, and Q is the quantity of fish offered for sale. Suppose there are n boats launched; then the quantity of fish caught is Q = naS. Fishers enter the market as long as profits are positive, which leads to zero profits for fishers; that is, $b=\left(\raisebox{1ex}{$Q$}\!\left/ \!\raisebox{-1ex}{$n$}\right.\right)p(Q)\text{.}$ This equation makes a company just indifferent to launching an additional boat because the costs and revenues are balanced. These two equations yield two equations in the two unknowns n and Q: $n=\frac{Qp(Q)}{b}=\frac{1}{b}{Q}^{\frac{\epsilon -1}{\epsilon }}\text{,}$ and Q = naS. These two equations solve for the number of fish caught, $,Q={\left(\frac{aS}{b}\right)}^{\epsilon }$ and the number of boats, $n=\frac{a^{\epsilon -1}}{b^{\epsilon }}{S}^{\epsilon -1}\text{.}$

Subtracting the capture by humans from the growth in the fish population yields

$$\dot{S}=rS(1-S)-{\left(\frac{aS}{b}\right)}^{\epsilon }\text{.}$$

Thus, a steady state satisfies $0=\dot{S}=rS(1-S)-{\left(\frac{aS}{b}\right)}^{\epsilon }.$

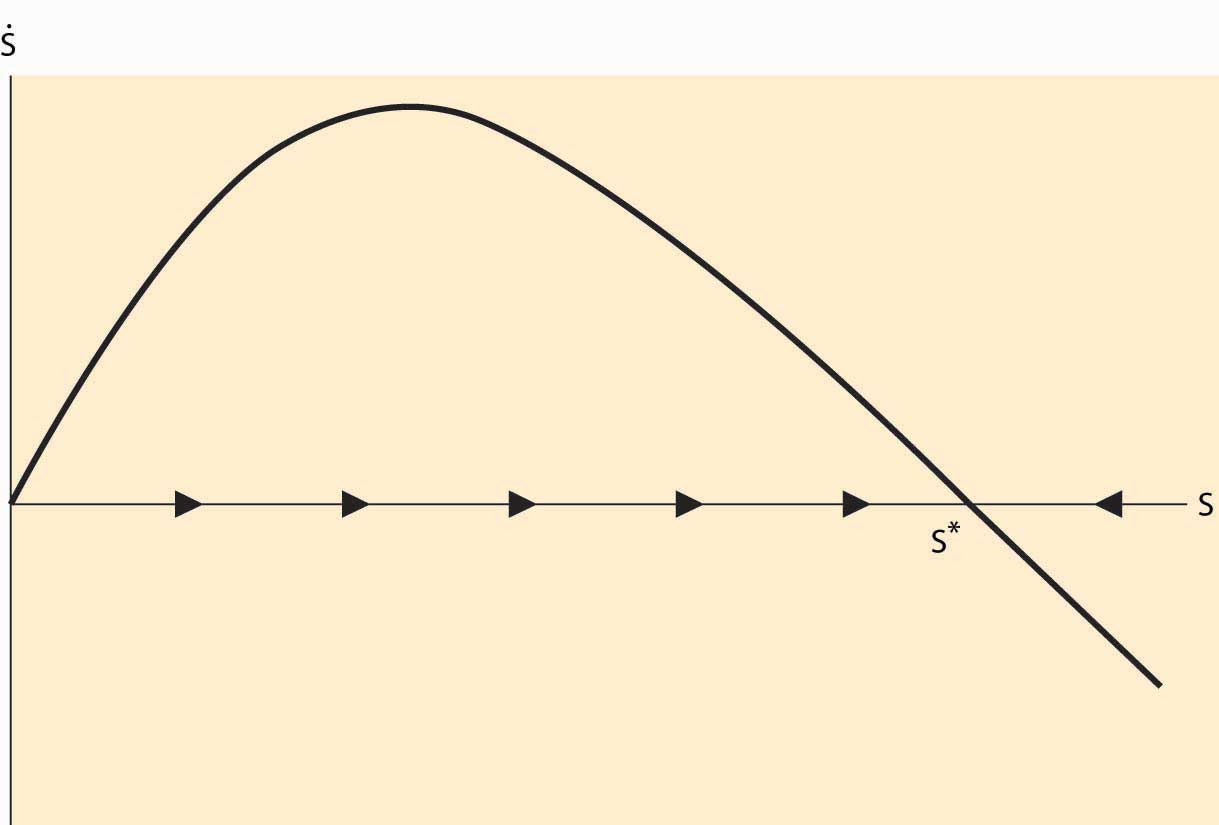
Will human fishing drive the fish to extinction? Extinction must occur when the only stable solution to the stock of fish is zero. Consider first the case when demand is elastic (ε > 1). Then, for S near zero but positive, $\dot{S}\approx rS>0\text{,}$ because the other terms are small relative to the linear term. Thus, with elastic demand, there is always a steady state without extinction. (Extinction is also an equilibrium, too, but overfishing won’t get the system there.) This equilibrium is illustrated in Figure 7.6 "Fish population dynamics with fishing".

The dark curve represents $\dot{S}$ , and thus for S between 0 and the point labeled S*, $\dot{S}$ is positive and so S is increasing over time. Similarly, to the right of S*, S is decreasing. Thus, S* is stable under small perturbations in the stock of fish and is an equilibrium.

We see that if demand for fish is elastic, fishing will not drive the fish to extinction. Even so, fishing will reduce the stock of fish below the efficient level because individual fishers don’t take account of the externality they impose—their fishing reduces the stock for future generations. The level of fish in the sea converges to S* satisfying

$$0=rS*(1-S*)-{\left(\frac{aS*}{b}\right)}^{\epsilon }.$$

In contrast, if demand is inelastic, fishing may drive the fish to extinction. For example, if r = 2 and a = b = 1, and ε = 0.7, extinction is necessary, as is illustrated in Figure 7.7 "Fish population dynamics: extinction".

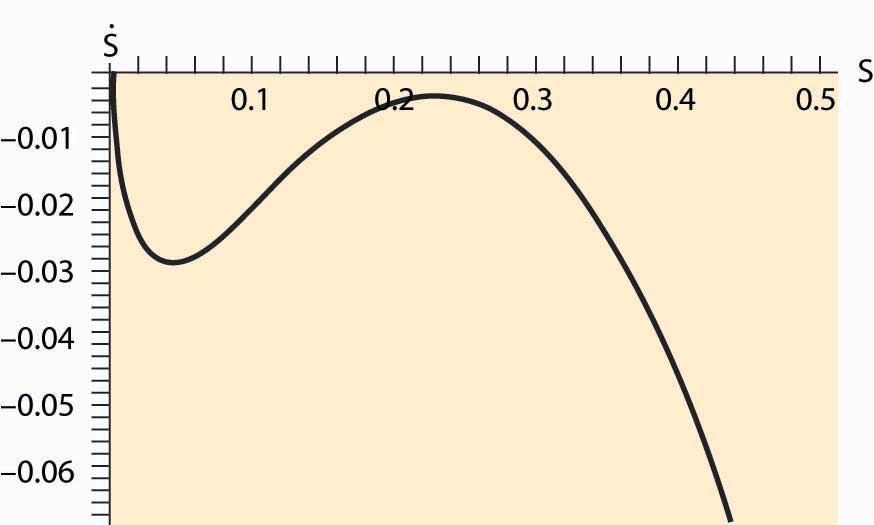
Figure 7.7 "Fish population dynamics: extinction" shows that, for the given parameters, the net growth of the fish population is negative for every value of the stock S. Thus, the population of fish consistently dwindles. This is a case when the fishing externality (overfishing today reduces the stock of fish tomorrow) has particularly dire consequences. The reason why the elasticity of demand matters is that, with inelastic demand, the fall in the stock of fish increases the price by a large amount (enough so that total revenue rises). This, in turn, increases the number of fishing boats, in spite of the fall in the catch. In contrast, with elastic demand, the number of fishing boats falls as the stock falls, reducing the proportion of fish caught, and thus preventing extinction. We see this for the equation for the number of fishing boats, $n=\frac{a^{\epsilon -1}}{b^{\epsilon }}{S}^{\epsilon -1}$ , which reflects the fact that fishing effort rises as the stock falls if and only if demand is inelastic.

It is possible, even with inelastic demand, for there to be a stable fish population. Not all parameter values lead to extinction. Using the same parameters as before, but with ε = 0.9, we obtain a stable outcome as illustrated in Figure 7.8 "Possibility of multiple equilibria".

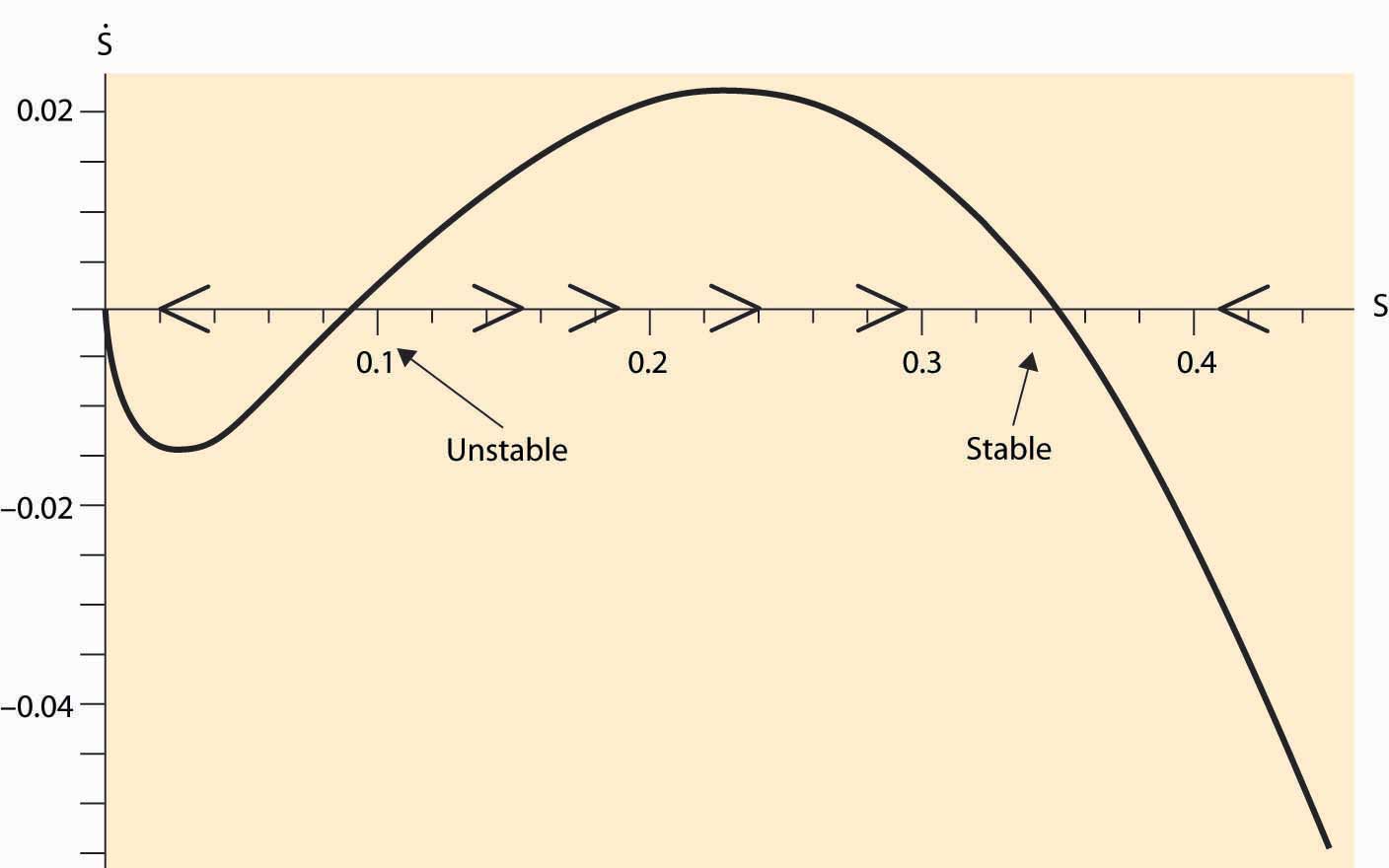
In addition to the stable equilibrium outcome, there is an unstable steady state, which may converge either upward or downward. A feature of fishing with inelastic demand is that there exists a region where extinction is inevitable because, when the stock is near zero, the high demand price induced by inelasticity forces sufficient fishing to ensure extinction.

As a consequence of the fishing externality, nations attempt to regulate fishing, both by extending their own reach 200 miles into the sea and by treaties limiting fishing in the open sea. These regulatory attempts have met with only modest success at preventing overfishing.

What is the efficient stock of fish? This is a challenging mathematical problem, but some insight can be gleaned via a steady-state analysis. A steady state arises when $\dot{S}=0\text{.}$ If a constant amount Q is removed, a steady state in the stock must occur at $0=\dot{S}=rS(1-S)-Q\text{.}$ This maximum catch then occurs at S = ½ and Q = ¼ r. This is not the efficient level, for it neglects the cost of boats, and the efficient stock will actually be larger. More generally, it is never efficient to send the population below the maximum point on the survival curve plotted in Figure 7.5 "Fish population dynamics".

Conceptually, fishing is an example of the tragedy of the commons externality already discussed. However, the threat of a permanent extinction and alluring possibility of solving dynamic models make it a particularly dramatic example.